Wetenschap
Nieuw model beschrijft trekjes, slakken en de rol van willekeur in overgangsturbulentie
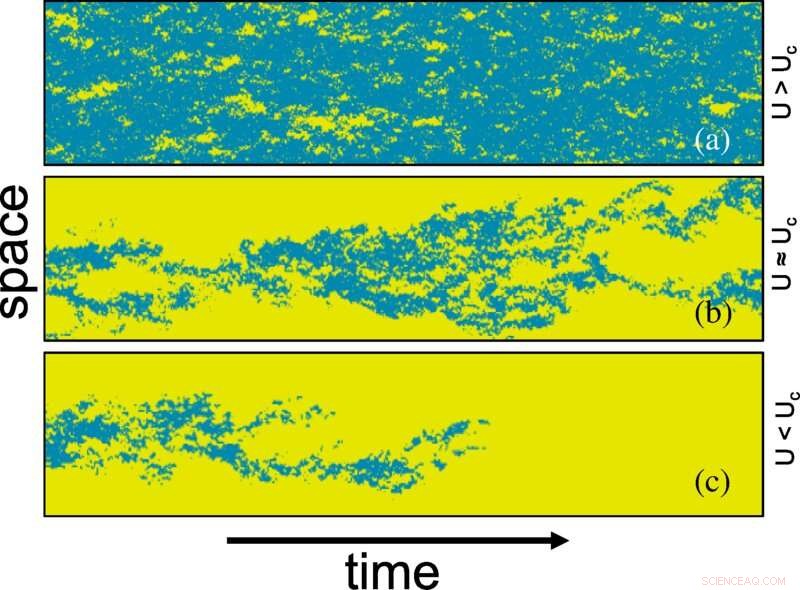
Ruimte-tijdplot van laminair-turbulente overgang als een functie van U (d.w.z. Reynolds-getal) gegenereerd door het PP-model in quasi-1D Taylor-Couette-stroom. Turbulentie (in blauw) wordt weergegeven door de prooidichtheid B, gegenereerd door Monte Carlo-simulatie op een 2D-rooster van 20×3000 (a) onder het kritische punt U=0.0135 , (b) op het kritische punt U=0.01425, en (c) boven het kritieke punt U=0,0165. Geel vertegenwoordigt de laminaire fase die lokaal niet door de prooi wordt ingenomen. De prooidichtheid wordt gebinariseerd naargelang deze groter is dan 0,065 × maximale prooidichtheid. Krediet:Fysieke beoordelingsbrieven (2022). DOI:10.1103/PhysRevLett.129.034501
Noem het woord "turbulentie" en je zou beelden kunnen oproepen van hobbelige vluchten, stormachtig weer en woelige oceaan- of rivierstromingen. Voor velen is turbulentie een feit van het dagelijks leven, maar het is ook een van de meest slecht begrepen fysieke verschijnselen. Met name het punt waarop de beweging van een vloeistof overgaat van een soepele en voorspelbare stroming (bekend als "laminair") naar willekeurig en onvoorspelbaar (bekend als "turbulentie") - de zogenaamde laminair-turbulente overgang - blijft wetenschappers sinds Osborne in verwarring brengen. Reynolds bestudeerde het voor het eerst experimenteel in pijpen in 1883.
Nu heeft een team van wetenschappers van de University of Illinois Urbana-Champaign, de University of California San Diego en Academia Sinica in Taiwan laten zien hoe de willekeurige patronen en dynamiek van turbulentie in leidingen in het overgangsregime kunnen worden verklaard. Hun werk maakt gebruik van nieuwe ideeën die hun oorsprong vinden in uiteenlopende gebieden als statistische mechanica en ecologie en het bouwt voort op het groeiende bewijs dat de laminair-turbulente overgang statistische eigenschappen heeft die het best kunnen worden beschouwd in termen van de theorie van niet-evenwichtige faseovergangen.
Het team bestaat uit UIUC-student natuurkunde Xueying Wang, Academia Sinica-onderzoeker Hong-Yan Shih en UIUC Swanlund Endowed Chair Emeritus of Physics and Research Professor Nigel Goldenfeld. Goldenfeld is momenteel de Distinguished Professor of Physics van de Chancellors' aan de University of California San Diego.
De auteurs publiceerden hun resultaten op 11 juli 2022 in het tijdschrift Physical Review Letters .
Rookwolken en slakken zijn kenmerken van overgangsturbulentie
Reynolds ontdekte dat in leidingen de laminair-turbulente overgang fragmentarisch plaatsvindt naarmate de stroomsnelheid toeneemt. Klodders turbulente vloeistof, tegenwoordig bekend als "rookwolken", verschijnen in de buurt van de laminair-turbulente overgang en worden gescheiden door gebieden met laminaire stroming. De precieze manier waarop de trekjes verschijnen en bewegen of zelfs in tweeën splitsen, hangt af van de geometrie van de ruimte waardoor een vloeistof stroomt. Deze complexe verschijnselen dragen bij aan de welverdiende reputatie van turbulentie als een van de laatste openstaande problemen in de klassieke natuurkunde. Bij nog hogere snelheden groeien turbulente plekken eigenlijk in plaats van alleen maar te bewegen of te splitsen:deze groeiende turbulentiegebieden worden 'slakken' genoemd.
Om een duidelijker beeld te krijgen van de overgang naar turbulentie, ontwikkelden de onderzoekers een nieuw minimaal model om trekjes en slakken te begrijpen met behulp van methoden die zijn geïmporteerd uit de theoretische populatiebiologie. De onderzoekers ontdekten dat ze de energiestroom van de vloeistof in de buurt van de laminair-turbulente overgang konden weergeven in termen van de energiestroom die ontstaat in een roofdier-prooi-ecosysteem, waarin voedingsstoffen de energie zijn van de achtergrondstroom, het roofdier is een bepaalde stroomstructuur die turbulentie remt, en turbulentie is de prooi. Dit ecologische model recapituleert turbulent gedrag in zowel pijp- als Taylor-Couette-stroming, een soort roterende stroming - een doel dat eerdere modellen niet konden bereiken.
Goldenfeld zegt:"Zes jaar geleden werd een doorbraak gemaakt met theoretisch en experimenteel bewijs dat samenkwam in een beschrijving van turbulente trekjes die voortkomen uit laminaire stroming, in termen van faseovergangstheorie. Dat werk liet echter de vraag open wat er gebeurt bij een hogere stroming. versnelt weg van het kantelpunt zelf.
"Ons nieuwe werk laat zien dat hetzelfde conceptuele kader en dezelfde methoden ook van toepassing zijn in het slug-regime en recapituleren in opmerkelijk detail de experimentele bevindingen. Het is fantastisch om concepten uit de faseovergangstheorie en ecologie samen te zien komen in het totaal andere probleem van de vloeistofmechanica. "
Naaktslakken vertonen zelf interessant gedrag en zijn er in twee smaken, zwakke slakken en sterke slakken, die beide worden gekenmerkt door ten minste één 'front', een gebied met een grens tussen laminaire en turbulente vloeistoffen.
Hoofdauteur Wang legt uit:"De voorkant van een slak is als een weerfront. Aan de ene kant van de voorkant is laminaire vloeistof. Aan de andere kant is turbulentie. De voorkant is als een fasegrens en beweegt in de ruimte met een constante snelheid. snelheid. In pijpen krijg je zowel slugs als puffs. Maar zwakke slugs bij lagere vloeistofsnelheid hebben slechts één front stroomopwaarts, terwijl sterke slugs bij hogere snelheid fronten hebben in zowel de stroomopwaartse als stroomafwaartse richting. Deze factoren en de rijkdom aan overgangsverschijnselen maken overgangsturbulentie heel moeilijk begrijpen. Ons werk biedt een uniform raamwerk dat al deze regimes, verschillende stroomgeometrieën en de inherente willekeur aankan."
Predator-prooimodellen en turbulentie komen samen
De onderzoekers konden profiteren van een verrassende verbinding die ze in eerder werk hadden gevonden tussen populatiebiologie en overgangsturbulentie.
Het modelleren van hoe roofdieren omgaan met hun prooi is een populair thema in de populatiebiologie. Het basisidee is eenvoudig:roofdieren reproduceren en eten prooien, waardoor hun populatie afneemt; prooien planten zich ook voort, herstellen hun aantal en voorzien roofdieren van voedsel. Dan herhaalt de cyclus zich. Simpel gezegd, roofdieren remmen prooien, terwijl prooien roofdieren versterken. Wetenschappers kunnen veel informatie uit deze modellen afleiden, zoals hoe roofdier- en prooipopulaties in de loop van de tijd variëren en hoe lang het duurt voordat ze uitsterven, bijvoorbeeld door een gebrek aan voedsel of overpredatie.
In een eerdere studie toonden Shih en Goldenfeld, in samenwerking met voormalig student Tsung-Lin Hsieh (nu een postdoctoraal onderzoeker aan de Princeton University), aan dat er een analogie bestaat tussen roofdier-prooimodellen en turbulentie die in wiskundige termen kan worden uitgedrukt. Wanneer vloeistof door een pijp stroomt, worden twee soorten vloeistofbewegingen gegenereerd. Het eerste type is een vortexpatroon dat rond de as van de pijp wervelt, "zonale stroming" genoemd. Het tweede type is turbulentie langs de pijpas. De auteurs ontdekten dat turbulentie gestaag accumuleert en zonale stroming activeert, die vervolgens turbulentie onderdrukt. Met andere woorden, zonale stroming komt overeen met roofdieren en turbulentie komt overeen met prooi.
De onderzoekers ontdekten dat de kansverdeling van de levensduur van tijdelijke turbulentie precies overeenkwam met die van roofdieren en prooien in een ecosysteem, een verbluffend verband gezien het feit dat populatiebiologie en vloeistofdynamica schijnbaar ongelijksoortige velden zijn.
Hong-Yan Shih comments, "This connection helps us understand the complex transitional behavior of turbulence from the point of view of phase transitions in statistical mechanics. Specifically, this discovery provides the key ingredients to construct an effective theory, which leads to the prediction that the laminar-turbulent transition in fluids is a non-equilibrium phase transition in the directed percolation universality class.
"Directed percolation can be thought of as the familiar process that happens when water drips through coffee grounds in a percolator. If the grounds are too tightly packed, water can't get through. On the other hand, if the grounds are too loosely packed, water can get through but the coffee is undrinkable. There's a critical point where the water just manages to get through and takes long enough so that the coffee tastes good.
"Mathematically, that phenomenon is exactly what happens in the transition to turbulence and the transition of a functioning ecosystem. The mathematics of phase transitions, founded in the Nobel Prize–winning work of K. Wilson, explains how this remarkable phenomenon arises."
This prior work, however, looked at the turbulence of a single puff. Real life isn't as simple, and real fluids near the laminar-turbulent transition contain multiple puffs that grow, die out, and interact in complex ways as the flow speed increases. The researchers needed to extend their model to capture more complicated dynamics beyond those of a single puff.
Extending the predator-prey model by incorporating nutrients
To capture the complex dynamics found in experiments beyond the critical point in the current study, the authors decided to take into account energy balance in pipe flow.
Wang explains, "Turbulence is a dissipative structure that needs constant energy input to be sustained, and that energy comes from the laminar flow. This fact was previously shown by exact computer simulations of the fluid equations, but did not allow us to understand in a predictive way the phenomena that would emerge."
The researchers realized that, just like zonal flow and turbulence require energy to persist, predators and prey need nutrients from their environment to survive.
"We wanted to make a minimal model of the full energy balance to extend the previous work and capture energy extraction of turbulence from laminar mean flow," Wang adds. "So we introduced another component into the 'ecosystem':nutrients, which represent the kinetic energy of the mean flow."
The researchers numerically simulated the extended predator-prey model on a two-dimensional lattice having a length much larger than its width. They watched what happens when nutrients—that is, laminar state energy, from the fluid dynamics perspective—flow into the ecosystem, are consumed by the turbulence, and are restored downstream of the turbulence.
The model maps several pathways for energy and population dynamics. The first pathway takes input energy from the energy budget to turbulent energy, like prey extracting nutrients from their environment. A second pathway takes turbulent energy to zonal flow energy, like predators eating prey.
Once they established these predator-prey-inspired pathways, they sat back and watched computer simulations based on the new model, wherein energy entered the pipe and passed through the pathways randomly. Out of the randomness emerged features of transitional turbulence such as puffs, slugs, and their associated fronts, reproducing results seen in experiments. The simulations showed that the appearance of puffs or slugs—and whether slugs are of the weak or strong type—is determined by the input energy (or equivalently, the speed) of the fluid flow.
The researchers then successfully reproduced all the transitional phenomena observed in pipe flow experiments and explained the underlying physics of puff splitting and growth. Specifically, they found that puff splitting and interaction is highly probabilistic. As the fluid speed increases, the probability of puff splitting also increases. The puff-slug transition is gradual, and it occurs when puffs split so frequently that they start to fill up the system densely.
Rotational flow and new questions for turbulence
In addition to pipe flow, the researchers also simulated a special type of rotational flow known as Taylor-Couette flow in which a fluid moves in the space between two concentric cylinders and the outer cylinder rotates relative to the inner one. Unlike pipe flow, where the energy enters from the high-pressure end of the pipe, Taylor-Couette flow is sustained by shear, the stress that occurs when two boundaries move parallel to one another. The new model easily incorporated this difference and reproduced the patterns of transitional turbulence seen in experiments, demonstrating the flexibility of the model.
"We showed that the rich and complicated dynamical features in transitional pipe flow can be understood with a simple three-level stochastic predator-prey model based on energy balance in pipe flow," says Wang. "Our model also works for quasi-one-dimensional Taylor-Couette flow. Since energy balance generally holds in fluid systems, we expect our model to be applicable to systems with more complicated geometries as well."
Having described turbulence broadly by implementing their model in two different geometries, the team is already looking to answer new questions.
Goldenfeld says, "The next challenge is to see if and how our probabilistic model can be extended to two- or three-dimensional flows. This problem has been intensely studied for well over twenty years, with new experimental data starting to appear."
For his part, Goldenfeld is pleased to see diverse techniques converge to solve problems in complementary ways, a beautiful demonstration of how different fields of science can inform each other.
Goldenfeld summarizes, "Our results show how stochastic dynamics, pattern formation, phase transitions, and modeling concepts from diverse fields such as ecology can bring new tools, predictions, and insights into a problem previously considered within the more narrow disciplinary focus of fluid dynamics. It is exciting to see how successful minimal modeling is at capturing complex physical phenomena in a quantitative way." + Verder verkennen
Ecological extinction explains how turbulence dies
 Wat zijn de voordelen van prokaryoten?
Wat zijn de voordelen van prokaryoten?  Diepzee-ontdekkingsreizigers vinden gezonken oorlogsschip uit belangrijke WO II-strijd
Diepzee-ontdekkingsreizigers vinden gezonken oorlogsschip uit belangrijke WO II-strijd Betalen voor emissies die we al hebben vrijgegeven
Betalen voor emissies die we al hebben vrijgegeven Blootstelling aan dodelijke stedelijke hitte is de afgelopen decennia wereldwijd verdrievoudigd, zegt studie
Blootstelling aan dodelijke stedelijke hitte is de afgelopen decennia wereldwijd verdrievoudigd, zegt studie Gigantische A-68 ijsberg drie jaar later
Gigantische A-68 ijsberg drie jaar later
Hoofdlijnen
- Cheerleading Science Fair Project Ideas
- eDNA gebruiken om de broedhabitat van bedreigde diersoorten te identificeren
- Drie manieren waarop genetische diversiteit optreedt tijdens meiose
- Hoeveel mieren zijn er op aarde?
- Wetenschappers onthullen de prachtige eenvoud die ten grondslag ligt aan vertakkingspatronen in weefsel
- Studie lost waarom drinken je de munchies geeft
- Thailand verbergt grote aantallen als het gaat om zijn visvangsten in aangrenzende wateren
- Nieuwe RNA-gebaseerde tool kan hersencircuits verlichten, specifieke cellen bewerken
- Bewijs dat energiemetabolisme en regulatie van biofilmvorming in bacteriën met elkaar verweven zijn
 Junior-onderzoekers strijden met succes voor extra NIH-beurzen
Junior-onderzoekers strijden met succes voor extra NIH-beurzen Quantum nanodiamanten kunnen helpen om ziekte eerder op te sporen
Quantum nanodiamanten kunnen helpen om ziekte eerder op te sporen Collegeklassen om een wiskundeleraar te worden
Collegeklassen om een wiskundeleraar te worden Studie naar dinosaurusveren ontkracht:overweldigend bewijs ondersteunt Jurassic-fossiel wel degelijk van Archaeopteryx
Studie naar dinosaurusveren ontkracht:overweldigend bewijs ondersteunt Jurassic-fossiel wel degelijk van Archaeopteryx Baanbrekende ontdekking bevestigt het bestaan van superzware zwarte gaten in een baan
Baanbrekende ontdekking bevestigt het bestaan van superzware zwarte gaten in een baan Wetenschappers ontwikkelen eerste 3D-massaschatting van microplasticvervuiling in Lake Erie
Wetenschappers ontwikkelen eerste 3D-massaschatting van microplasticvervuiling in Lake Erie Is straf wel zo effectief als we denken?
Is straf wel zo effectief als we denken? 'S Werelds kleinste optische implanteerbare biodevice
'S Werelds kleinste optische implanteerbare biodevice
- Elektronica
- Biologie
- Zonsverduistering
- Wiskunde
- French | Italian | Spanish | Portuguese | Swedish | German | Dutch | Danish | Norway |

-
Wetenschap © https://nl.scienceaq.com